Vector Addition Goodness - and Quirkiness

This went well.

It turned out to be a quick way to generate lots of examples - simple ones and quirky ones, and to target specific examples so that we could look back and look ahead in the vector unit.

We've just started vector addition. Here's the activity I put together for my online class today:

We're all online, so anyone can easily move these arrows around by clicking and dragging them.

I split the kids into groups of 2-3, and told them to each pick one blue and one green vector, add them, then draw the resultant in red (these colours matched the ones from the previous evening's voicethread.). Since there are 6 blue and 6 green, there are many possible combinations of vectors - 36 in fact (an opportunity to talk about math that's not usually part of the vectors unit!). They didn't have to do them all, of course, just do 3 pairs, or enough to use up all the vectors.

Because they were dragging instead of drawing, it only took about 5 mins for them all to finish. I took snapshots of each group's work, then we reconvened in the main classroom space. Here were some of the results

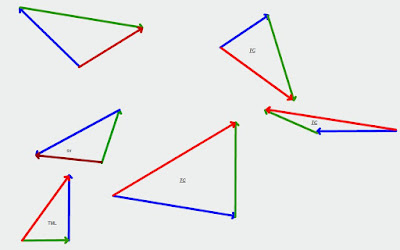
Group 1:

First we together looked at all the examples group 1 made and decided if there were any mistakes - which happily, there weren't. Then I saw the first part of my evil plan unfold. (I had only a 1/36 chance of that combo happening, yet it happened!) The combo at the very lower left was two horizontal vectors of opposite directions being added. A quirky one that I wanted everyone to see, and since only one group did that combo, it kind of was an example that had a personality to it - it belonged to a person!

Group 2:

We repeated for group 2 - checked the answers first, then more evil plans unfolded. (Another long shot happened, geez I should buy a lottery ticket today.) In the lower left corner, someone had added a green and blue that were perpendicular to each other. I asked them to think about where they'd seen something like that before - and after a few seconds I heard - the blue and green are almost like the components of the red. Almost? OR EXACTLY?!? We talked about how all along, the components of a vector (with which they're already familiar) actually add up to that vector. When they'd been drawing a vector's components all last week, it was kind of like they started with the answer, and drew a question to go with it.

Group 3:

Weird how all my evil combos ended up in the lower left corner...anyway here we saw the same component-style example except that they're added in a different order, but still giving the same resultant.

I then showed them a combo that no one had picked, but that I wanted to address:

I had, of course, deliberately put in a blue and green that were identical to each other. We talked about what the resultant for this would look like, and it was a nice intro to something we haven't done yet - multiplication of a scalar and a vector.

What I liked about this activity:

• the possibility of everyone seeing so many examples in a relatively short time (this all took a total of 20 minutes)
• the possibility of interesting and unusual examples for discussion
• the personalization of the examples - I didn't make them up, not really anyway, so that I could refer to "Susie's example", instead of a cold "number 3"
• It gave kids who think outside of the box a chance to try something outside of the box, and feel like it's ok to do that. In fact, it was fantastic to do that!
• It was a great intro to the geogebra that I then had them do individually, in which they were pretty much doing the same thing, manipulating vectors to add them, with a few twists, like find what vector I have to add to this one to get that one (intro to subtraction)

Next time:

• I'll make sure to keep WAY more copies of the original arrows - easier to show combos that no one did that way
• Find cases where two people added the exact same vectors and got the exact same resultant
• I'll include the potential for more quirkiness, like opposite vectors adding to zero. You can never have too much quirkiness.