Honestly, what with my withering attention span and
emotional ups and downs, it’s amazing that I manage to get anything done. But I do nevertheless, and this is what I managed to get done on the afternoon of this past Valentine’s
Day. I needed to write about it because it was kind of awesome, and because it gave me some insight into my students' reflections in their eportfolios.
What happened is, I inadvertently went on a quest, in my head. The journey itself was fascinating, at least in retrospect, because it seemed like a meta-cognitive experience. Since it's probably the kind of thing I'm supposed to be getting my kids to do and write about in their eportfolios, it's very fortunate that I did it myself, and apropos that I write about it too. And although they say that the journey is more important than the destination, it was pretty sweet when I got there.
What happened is, I inadvertently went on a quest, in my head. The journey itself was fascinating, at least in retrospect, because it seemed like a meta-cognitive experience. Since it's probably the kind of thing I'm supposed to be getting my kids to do and write about in their eportfolios, it's very fortunate that I did it myself, and apropos that I write about it too. And although they say that the journey is more important than the destination, it was pretty sweet when I got there.
What was the problem?
This little paragraph in our textbook that has tormented me for many years:
This little paragraph in our textbook that has tormented me for many years:
The rule of a logarithmic function can be written in the form f(x) = alogcb(x - h) + k. However, certain algebraic manipulations allow you to transform this rule and write it in the standard form f(x) = logcb(x - h).
To worsen the torment, I've said to my students "Right. Sorry. I don't know what those certain algebraic manipulations are, but it says here that they exist and they work, so let's just go with it, shall we?" And another little piece of my self-respect as a teacher snapped off.
Anyway, I had a ton of other things I was supposed to be doing,
so naturally, I started playing with the expression
to try to disappear the a and the k. I just kind of fell into it, just like that. Kind of like Frodo.
And so began the quest:
(There is math here, but I've tried to intersperse it with enough other stuff that anyone who's ever tried to figure something out might be able to identify with it.)
Anyway, the k part was easy, so I started with that. Easy, I just replaced the k:
+%7B%5Ccolor%7BRed%7D&space;k%7D=log_%7Bc%7Db(x-h)+%7B%5Ccolor%7BRed%7D&space;log_%7Bc%7Dc%5E%7Bk%7D%7D)
At this point, I couldn't help but notice that that last term, in red, looked and sounded a lot like Louis CK, so I watched a few of his hilarious videos. Very productive.
(Approximately a half hour later): The whole reason I did that last step was in preparation for this next one, in which we...
Smoosh the logs::
%7D+log_%7Bc%7D%7B%5Ccolor%7BRed%7D&space;c%5E%7Bk%7D%7D=log_%7Bc%7D%7B%5Ccolor%7BRed%7D&space;b(x-h)c%5E%7Bk%7D%7D)
Normal people would call this last step "applying the first property of logarithms" , or, "because the sum of the logs is the log of the product", but I and my students call this "smooshing the logs." (Sorry, former and current students, for using vocabulary that no one else recognizes or takes seriously. But you had fun, am I right?)
So far, I had managed to get rid of the k by combining it with the c and the b to form what's in red:
+k=log_%7Bc%7D%7B%5Ccolor%7BRed%7D&space;bc%5E%7Bk%7D%7D(x-h))
But that was very routine, baby stuff, nothing new. In fact, I'd gotten this far in previous years. The real rub was what to do with that darn "a". I was convinced that I could only get rid of it by applying another property in an equally routine and unoriginal way:
And so began the quest:
(There is math here, but I've tried to intersperse it with enough other stuff that anyone who's ever tried to figure something out might be able to identify with it.)
Anyway, the k part was easy, so I started with that. Easy, I just replaced the k:
At this point, I couldn't help but notice that that last term, in red, looked and sounded a lot like Louis CK, so I watched a few of his hilarious videos. Very productive.
(Approximately a half hour later): The whole reason I did that last step was in preparation for this next one, in which we...
Smoosh the logs::
Normal people would call this last step "applying the first property of logarithms" , or, "because the sum of the logs is the log of the product", but I and my students call this "smooshing the logs." (Sorry, former and current students, for using vocabulary that no one else recognizes or takes seriously. But you had fun, am I right?)
So far, I had managed to get rid of the k by combining it with the c and the b to form what's in red:
But that was very routine, baby stuff, nothing new. In fact, I'd gotten this far in previous years. The real rub was what to do with that darn "a". I was convinced that I could only get rid of it by applying another property in an equally routine and unoriginal way:
Normal people: Apply the third property of logarithms.
Me and my students: Actually, that's what we'd say. Sometimes I model normal behaviour to keep them guessing.
But the only thing I could think of to do next was this really lame exponent thing:
On Valentine's Day, for some reason, that (x - h) with the exponent of "a" sorely vexed me.
The Cycle of Distraction and Torment
I then began a cycle, which I repeated many times that afternoon,which went a little like this:
I'm afraid I became a bit crazed. I'm looking right now at all the papers I scribbled on furiously. Here's one of them:
The Turning Point
It wasn't that I finally knuckled down, or suddenly discovered a new math thing, or gained insight from geogebra. The turning point came about when myself talked to myself. I literally heard my brain say to me "You're not thinking like a logarithm. Think like a logarithm. BE an exponent."
Then this actual logarithm idea kept popping up, something I remembered noticing a long time ago. An idea that wasn't terribly difficult, but it had been of my own making:
If I say that:
that is, they both equal 9, I'm really saying that the exponent that 3 needs to turn it into a 9 is the opposite of the exponent that 1/3 needs to turn it into a 9. In log language, that's:

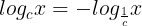
How to think like a logarithm:
Now this didn't solve my problem, but it gave me a hint about how I needed to think. Stop mindlessly applying routine algebraic procedures, and think about what a log is, how it behaves, and how logs with different bases can be related.
Or more generally:
How to think like a logarithm:
Now this didn't solve my problem, but it gave me a hint about how I needed to think. Stop mindlessly applying routine algebraic procedures, and think about what a log is, how it behaves, and how logs with different bases can be related.
Now I saw "a" as a number multiplying an exponent, and I played around with this kind of thing:
but if I change my base to 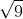 , or 3, and still want to get 729, then it's:
, or 3, and still want to get 729, then it's:
%5E%7B%7B%5Ccolor%7BRed%7D&space;2%7D%5Ccdot&space;3%7D=729)
I'll spare you the details of my further number experiments, for there were many, and they were feverish, but the important thing is this:
At this point, there was nothing on this earth that could have distracted me.
Or even discouraged me. I was possessed. I still didn't know the answer, but I knew I was on the very verge of getting it, and now that I look back, this was the most exhilarating part. I owned the problem, and I knew in my bones that it was just a matter of time before I'd get it. I was actually shaking a bit.
I can count the number of times this has happened to me on the fingers of two hands. Maybe that's the way it's supposed to be, after all, it was pretty exhausting! (The last time it happened, I wrote about it here. Projectiles. Enough said.)
Once I was sure of the numbers, I put it all down algebraically to summarize. It occurs to me that this is the most boring part, because it's like a synopsis of a story, rather than the actual story. And usually this is the part we show our students, the boring synopsis!
The math behind the "a", if you're interested:
The math behind the "a", if you're interested:
But then:
which means that
which, put logarithmically, is:
See above in red, by which I can replace n:
which told me I can get rid of the "a" by combining it with the c to form a new base, c ^(1/a).
After certain algebraic manipulations
and much checking and re-checking with geogebra, this thing of beauty finally revealed itself to me:
which I immediately tweeted, and to which my wonderful brilliant friend Jennifer Silverman immediately replied::
which I immediately tweeted, and to which my wonderful brilliant friend Jennifer Silverman immediately replied::
@a_mcsquared You are SUCH a romantic, my dear!
— Jen Silverman (@jensilvermath) February 14, 2014
What happened then?
Well, I shared it with my students the following week, just so that I could have the satisfaction of NOT losing another little piece of my self-respect as a teacher for once. Their reactions could be summarized as "Do we need to know this?". But I also shared with them the journey that had lead to it, and I think that made way more of an impression. I hope what they'll remember is:
- No one can ever say they know all there is to know about something.
- You can never call anything you know useless, because you never know when you'll need it.
- Your brains are miracles, not because of the facts they can hold, although that is pretty amazing, but because it can solve problems while you're not even aware of it.
- You can actually influence how you think!
- Everything that I ask you to do I'll do too. Including and especially, keep learning.





Brava!
ReplyDeleteThanks my friend! I hope your Valentine's day was good too!
DeleteTOTALLY impressed. Loved the conversations you had w/ yourself, especially the part about "This is taking too much time and I have real work to do...." (or something like that: it would take me too long to scroll back up and find it), but then you go to, "Oh wait, what if I try...." First, this models persistence which is what we need to teach kiddos. Second, it was like listening to myself. It made me laugh right out loud. Not to mention that you saw Louis CK, too! Seriously, are we twins separated at birth or what!!? Nicely done!
ReplyDeleteThanks so much Tina! it means so much to me that anyone reads to the very end (my posts tend to be long), likes it, laughs, and comments. Wow! We must have been SAB, bc that Louis CK jumped right out at me too. Tks again!
DeleteWow. This post is awesome for a few reasons...
ReplyDelete-Noticing the issue in the first place. Most wouldn't have thought much of the 'a' in the exponent, let alone dealt with it, let alone then blogged.
-The way you decided to go through the whole thought process, up to and including rough notes. (I think the video was superfluous though! You carry this post, particularly in light of...)
-The little headshot graphics. I don't know, for some reason I find that funny, in a good way, in how you linked them to bubbles. I imagine pasting the math graphics into the right places was something of a pain too.
-The lesson you finally built up to. The logs themselves were really just the vehicle. Nice.
Amusingly (and a bit egocentrically, sorry), this is exactly the sort of thing I credit my math personifications with - giving people a little nudge in the right direction and letting them go nuts, as it were. So "Thinking like a Logarithm" indeed! Logan approved! Here's hoping you feel that feeling you felt more often.
Thank you for this logtastic comment!
DeleteI wondered about the video. I have some friends who never read my blog because they can't understand anything in my posts, it's all too technical and mathy, so maybe that video was for them.
The headshots are all in a file for just such occasions, although they're all leftover from a video that was made from one of my posts, the Explainaholic one, but the callouts, yeah they were a bit of a pain. But that's also part of the story, getting this post done right also consumed me, with details like that. Thanks for noticing!
And the math graphics were easy actually, I just used codecogs.org. It generates the html for your math expression, and you just paste it into the html editor. Listen to me. A couple of years ago I wouldn't have said any of that.
I'm still feeling the feels, but now it's even better because I've shared it and you as usual just get me right away. I'm in meta-cognition logtopia!